This kind of information often proves to be extremely valuable, especially in the early stages of a research project. The analysis shows that, other things being equal, the drag force will be proportional to the density of the fluid. The analysis also gives other information for free, so to speak. Consequently when a body is moving relative to a gas, the drag coefficient varies with the Mach number and the Reynolds number. The Buckingham pi theorem then leads to a third dimensionless group, the ratio of the relative velocity to the speed of sound, which is known as the Mach number.
These two properties determine the speed of sound in the gas at its given temperature.
Did you get the dimension for kinematic viscosity C. The dynamic viscosity of a medium divided by its density is called its kinematic viscosity symbolized by the Greek letter u (nu)L 2 /T.
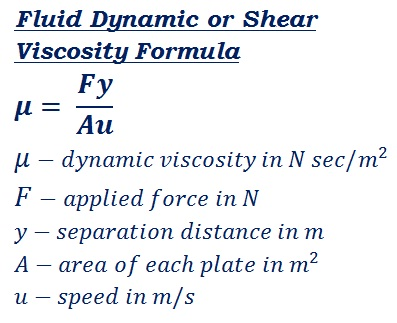
Those properties are conventionally considered to be the absolute temperature of the gas, and the ratio of its specific heats. The Greek letter µ (mu) stands for the dynamic viscosity of the medium M/(LT). If the fluid is a gas, certain properties of the gas influence the drag and those properties must also be taken into account. Thus the force is simply ½ ρ A u 2 times some (as-yet-unknown) function f c of the Reynolds number Re – a considerably simpler system than the original five-argument function given above.ĭimensional analysis thus makes a very complex problem (trying to determine the behavior of a function of five variables) a much simpler one: the determination of the drag as a function of only one variable, the Reynolds number.